Assignment: 06-Properties of Equality
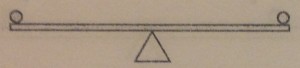
This lesson takes a closer look at that word equal and what it really means. We can think of an equation much like you would a scale where the middle of the scale is the equal sign. Let’s start with 1=1.
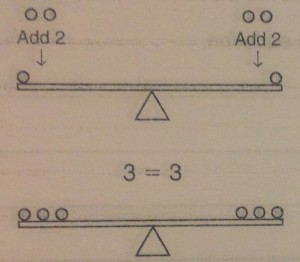
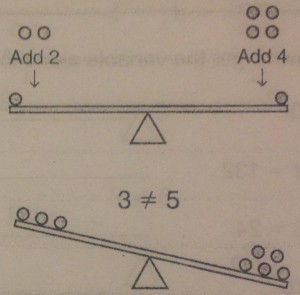
Currently the scale is balanced. We can make changes to that scale as much as we want, so long as we remember that whatever we add or subtract from one side, we have to do the exact same to both.
The most important part of this is remembering that bit. Whatever you do to one side, has to be done to the other, otherwise the expression is no longer equal.
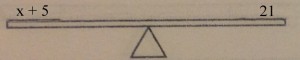
 We use these properties of equality as we start moving into algebra as it is the key to keeping equations equal while solving for the variable. Let’s say we have the problem x+5=21, and we have been asked to figure out what x equals. To do this, we need to get the variable alone. We could think of this on the same scale.
We use these properties of equality as we start moving into algebra as it is the key to keeping equations equal while solving for the variable. Let’s say we have the problem x+5=21, and we have been asked to figure out what x equals. To do this, we need to get the variable alone. We could think of this on the same scale.
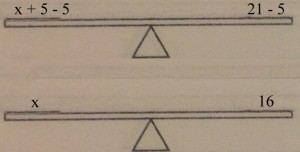
To get the variable alone, we need to get rid of the +5. The two key words are “opposite operation”. To get rid of a term like that we need to put it’s opposite on the scale. The opposite of +5 is -5. Remember though, whatever we do to one side, we have to do to both sides.
We’ve decided that x=16. We could then go back and check our answer by putting 16 in for the x in the original equation. 16+5=21 is true, so our answer is correct. These same rules apply for all operations, so knowing the opposites is one of the most important parts of this lesson. The opposite operations are addition and subtraction, and multiplication and division.