In my opinion, multiplying is the easiest operation to handle with fractions. There’s 3 simple steps:
1. Multiply the Numerators
2. Multiply the Denominators
3. Simplifiy
Here’s an example:
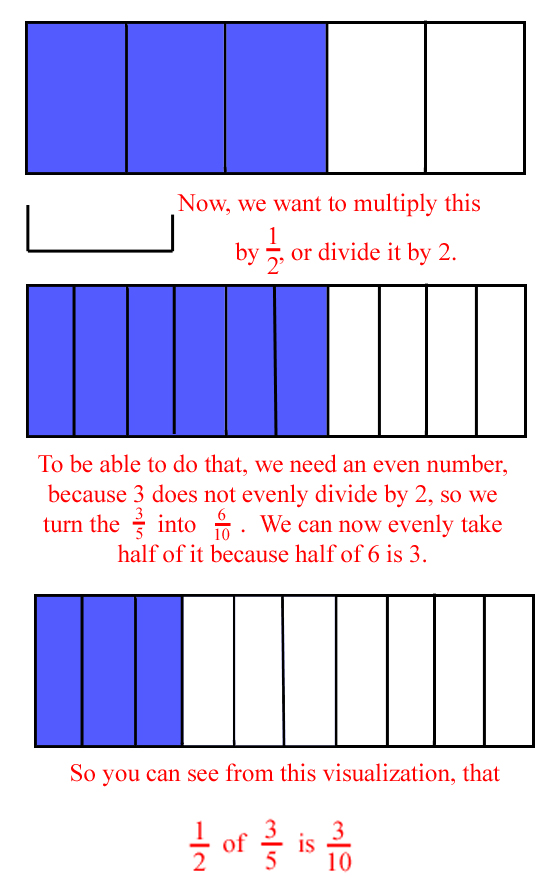
Another way to think about multiplying by a fraction, is to think about dividing by it’s reciprocal. A reciprocal is just the inverse of a fraction, so with the one-half above, it’s reciprocal would be 2 so
Here’s a visual representation what this would look like.
The more complex the fractions, the harder it is to visualize, but the concept is the same. Multiply the top, multiply the bottom, simplify.
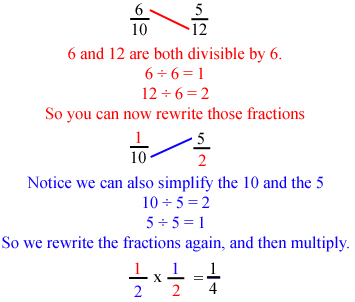
Now it is possible to simplify before multiplying, which to some is easier than simplifying after. You look at the numbers that are diagonal from each other, so in this case, the 6 and the 12, and the 10 and the 5. If there is a common factor, you can divide them down before multiplying to simplify the multiplication as well.
Notice, that even though we simplified before, our results were same as when we simplified after.
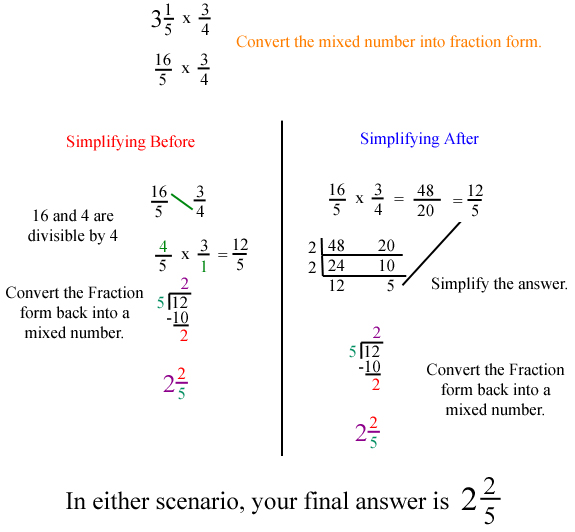
The only other thing we now throw into the mix, is mixed numbers. Remember these are when we have a whole number and a fraction. You have to convert the mixed number into its fraction form, and then, the math is the same.