Topics
1. Unit 3 Pretest
2. Introduce and Simplify Fractions and Mixed Numbers
3. Add & Subtract Fractions
4. Add and Subtract Mixed Numbers
Introduce and Simplify Fractions and Mixed Numbers
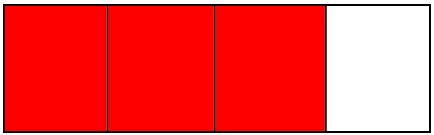
This unit begins by reviewing what a fraction is, which shouldn’t be too difficult, as they’ve been working with fractions for a few years now. However, we take a day to bring it back up anyway. In this first lesson we spend just a little time reviewing that a fraction is just a part of a whole number. If you have 3/4, then you could represent it by picture as shown below:
Next is to make sure they understand the proper terminology for the parts of the fraction. Below is a mixed number (a fraction with a whole number) with the parts of it labeled.
We will also talk about converting mixed numbers to fraction form and vice versa. To convert a mixed number to its fraction form you have to multiply there are three steps:
1. Multiple the whole number by the denominator
2. Add the numberator
3. Put that sum back over the original denimonator
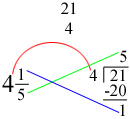
Fraction form to mixed number involves these steps:
1. Divide the numerator by the denominator.
2. The quotient is your whole number
3. The remainder is your numerator
4. Keep the original denominator
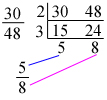
The final thing we will work on is simplifying. Now, I would like to mention that one of the pushes with the common core is less on the idea of simplest form, and more on the idea of equivalent fractions, so I will cover both here. For simplest form, you have to find the GCF of the numerator and denominator, and then divide them by their GCF. The beauty of the cake method to find the GCF, is that it does this for you. Example:
Equivalent fractions are found simply by either multiplying or dividing the numerator and denominator by the same number. If we take the fraction from, the equivalent fraction to the left is divided by 2, the fraction to the right is multiplied by 2. All three fractions are equivalent.
The easiest way to know if two fractions are equivalent is to take them both to simplest form. Example:
Add and Subtract Fractions
When adding or subtracting fractions with the same denominator, you simply add or subtract the numerators and keep the denominator. Examples:
If you have unlike denominators, then you need to find a common denominator. If the greater denominator is a multiple of the lesser, than you only need to adjust one fraction. You change it multiplying the denominator by whatever you need to make it the same. Whatever you multiply the denominator by, you have to also multiply the numerator by to keep it an equivalent fraction. The example is addition, but subtraction works exactly the same.
If the greater denominator is not a multiple of the lesser, than the easiest way to convert them to a common denominator is by multiplying one fraction by the other denominator and vice versa. Remember, we have to multiply both the numerator and the denominator to keep them equivalent. The example is subtraction, but addition works the same.
The last step I show there, the ones circled in green, is showing taking the answer and converting it into its simplest form.
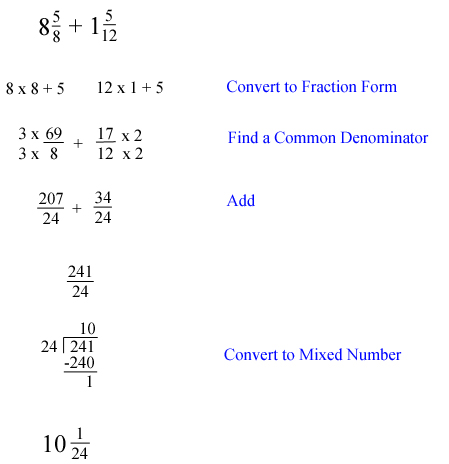
Add and Subtract Mixed Numbers
The only step added to this as compared to fractions is converting the mixed number into its fraction. Other than that, the steps are exactly the same. The fraction form of the answer is correct, however, they may often times ask to convert it back into its mixed number.


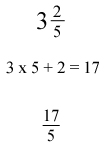


Pingback: Math Week 11 | Mr. Pratt's 6th Grade Class